Making a miniature hot air balloon (not)...
So I decided to run a small feasibility study. The objective here was to see if making a small hot air balloon from common household materials such as bin liner and birthday candles could yield something small enough to gain buoyancy indoors.
As this has become a rather long post, I'll divide it into chapters (the links will take you to the relevant sections):
1. Hot air balloons and Archimedes.
2. A practical example.
3. Short-cut for non-geeks.
4. Conclusion.
5. A few mini hot air balloons.
1. Hot air balloons and Archimedes.
d x V x g (1)
where g is the gravitational acceleration (approx. 10 m/s2, meter per second squared).
The object with a mass m (g, gram) will of course also experience a downward gravitational force, i.e. its weight:
m x g (2)
If the upward force caused by the displacement of the surrounding fluid exceeds the gravitational pull-down, the object will float, so:
d x V x g = m x g (3)
or simply: d x V = m (4)
In the case of the hot air balloon the density of the fluid is da, the density of ambient air (in g/l). V is the volume of the balloon (in l) and m the mass of the balloon (in g).
It's important to note the mass of the balloon is made up of two components: the mass of the equipment, me (in g), including the basket, burner, balloon material, payload etc on the one hand, and the actual weight of the hot air contained in the balloon, mh (in g), on the other hand.
Equation (4) then becomes:
da x V = me + mh (6)
The mass of the hot air contained in the balloon can be calculated from the Ideal Gas Law, p x V = n x R x T (7) where p is the pressure of the air in the balloon (in atm, atmosphere), n the number of moles of air contained in the balloon, T the temperature of the air in the balloon (in K, Kelvin [Kelvin = Centigrade + 273]) and R the ideal gas constant (0.082 atm.l/mole.K, atmosphere times litre per mole per Kelvin).
With a bit or reworking (7) yields the mass of the hot air contained in the balloon:
mh = p x Mair / (R x T) (8)
Here Mair is the molecular weight of air (approx. 29 g/mole, gram per mole). Factoid: the molecular weight of air is approximately the weight of 1 trillion trillion air molecules (6 x 1023, or 600,000,000,000,000,000,000,000 to be more precise)!
Inserting (8) in (6) and a little more reworking yields:
T = p x Mair x V / [R x (da x V - me)] (9)
Here T is the minimum temperature (in K, Kelvin [Kelvin = Centigrade + 273]) the hot air inside the balloon has to reach for lift-off to occur.
We can simplify this equation by stating:
α = p x Mair / R (10)
Note that in normal conditions (room temperature and atmospheric pressure) α is a constant and approx. 358 (K/l, Kelvin per litre).
And:
β(V) = V / (da x V - me) (11)
Please note that whereas α is a constant, β(V) is not and is a function of the balloon volume V, hence the notation β(V) (β, function of V).
Equation (9) then becomes:
T = α x β(V) (12)
The function β(V) is worth exploring because it shows both a vertical asymptote and a horizontal asymptote.
The vertical asymptote occurs at Vmin = me / da (13), where the denominator of β(V) becomes zero and β(V) = ∞ (infinity!) This isn't just a mathematical quirk of β(V), no, this asymptote has real physical meaning. It means that the balloon needs a minimum volume Vmin = me / da or else it can never become airborne, no matter what the temperature of the air inside the balloon is. In fact, even at Vmin = me / da, the temperature of the air would still have to be infinitely high, because only then would the mass of air inside the balloon, mh (from (8)), become zero and (very slow) lift-off would occur. (Back to top.)
2. A practical example.
Let's take a practical example. I got the flimsiest plastic carrier bag possible (I recommend Spar shops for this!) and four birthday candles (as "burners"), which gave me a total weight me of 10 g (gram). I didn't measure the volume V of the bag but estimated it to be somewhere between 5 and 10 l (litre).
Now you would think it would be possible to get such lightweight thingy up in the air without too many problems but you'd have thought wrongly. Let's check out (13) with some numbers. The density of dry air at 25 Centigrade is approximately 1.2 g/l (gram per litre), so (13) becomes Vmin = 10 g / 1.2 g/l = 8.33 l. So we'd have a Spar shopping bag of 8.33 l, fired by four birthday candles, getting buoyant when the air inside has reached infinitely high temperature... pardon the pun but that's not going to fly! The balloon in other words needs to be larger to stand a chance.
Here are a few examples for me of 10 g and various volumes and the hot air temperature required for lift-off:
Volume (l) >>>>>>>>>>>> Temperature (Centigrade)
10 >>>>>>>>>>>>>>>>>> 1,500
15 >>>>>>>>>>>>>>>>>> 400
20 >>>>>>>>>>>>>>>>>> 240
25 >>>>>>>>>>>>>>>>>> 175
50 >>>>>>>>>>>>>>>>>> 85
Attentive readers may have noticed that there is a bit of a contradiction going on as the balloon weight me cannot be expected to stay constant with ever increasing volume V of the balloon. In fact, it can be shown that in most cases the balloon mass must be a function of V, that is me(V), which is probably directly proportional to V raised to the power 2/3, so something like me(V) = k x V2/3, with k a material constant. This makes the whole scenario even worse as the temperature of the hot air will have to be even higher to compensate for the extra weight for larger balloons but it doesn't invalidate the principles outlined here.
Looking at the horizontal asymptote at β(V) = 1 / da, this simply indicates that for an infinitely large balloon (V = ∞), T∞ = 25 Centigrade. (Back to top.)
3. Short-cut for non-geeks.
For those who find the algebra and physics too nerdy or are still sceptical about the non-feasibility of a truly miniature hot air balloon, try this short-cut. The birthday helium balloon (V about 10 l) that inspired this post was buoyant because the helium has a density of about 0.18 g/l (at room temperature). To reach the same buoyancy by replacing the helium with hot air, the air would have to be hot enough for its density to reach the same value. The density of dry air can be calculated simply from dh = 358 / T (in g/l), so for dh to become 0.18 g/l, T would have to be 1,715 Centigrade (1,990 K)! Had the balloon been filled with hydrogen gas (see also the Hindenburg disaster) with a density about 0.09 g/l at room temperature, the balloon would have been even more buoyant and the air to replace it would have to have been at 3,700 Centigrade (3,980 K)! (Back to top.)
4. Conclusion.
In short, miniature hot air balloons are possible but aren't exactly miniature. From around 50 litres or so a functional hot air balloon becomes feasible but it's not something you could run indoors: use... good old helium balloons for that. (Back to top.)
5. A few mini hot air balloons.
Here are some Frenchmen and their toy hot air balloons. Note the size of these babies!
Here's another homemade mini hot air balloon, this one twice as high as a child...
This here "crafts enthusiast" is full of hot air but manages (not) to make a "hot air balloon" with no heat source in sight. It's just a dangling balloon, dear...
(Back to top.)
Finally, here's a miniature self-combusting "hot air balloon": sorry to burn your balloon...
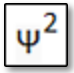
6 Comments:
That was over my head, the equations, not the balloon.
Me, I love a little algebra in the morning...
Gert mate....you used formula's and equations that I have not used, nor even thought of for many, MANY years. There was a time that I could have followed your presentation to the end, and completely understood everything...but alas...not at this time. I give you credit fer keep in yur mind sharp by utilizing all the principles and math involved.....oh well...up, UP and Away....
Cookie:
There's an advantage to being a geek, I guess...
Reminds me of the Irish space rocket I was shown at a dinner party once. It started with a tea bag and an amusing spiel about the Irish trying to build a space rocket, but deciding fuel was too heavy (out goes the tea leaves) and other necessary things I don't remember, but caused the ticket and the then the string to go away with a snip of the scissors,and the resulting empty paper bag, held over the candle, went up with a sudden, delightfully surprising whoosh. The rocket worked! Sadly, I seem to recall that it also burned up. But it made a pretty spark!
Morva Shepley
great post; hot air balloons are truly fascinating. Thanks for sharingo your experiment
Post a Comment
<< Home